(Continued from April 2013 and later)
This is what I called "the large Desargues configuration"
in posts of April 2013 and later.
(Continued from April 2013 and later)
This is what I called "the large Desargues configuration"
in posts of April 2013 and later.
Desargues' theorem according to a standard textbook:
"If two triangles are perspective from a point
they are perspective from a line."
The converse, from the same book:
"If two triangles are perspective from a line
they are perspective from a point."
Desargues' theorem according to Wikipedia
combines the above statements:
"Two triangles are in perspective axially [i.e., from a line]
if and only if they are in perspective centrally [i.e., from a point]."
A figure often used to illustrate the theorem,
the Desargues configuration , has 10 points and 10 lines,
with 3 points on each line and 3 lines on each point.
A discussion of the "if and only if" version of the theorem
in light of Galois geometry requires a larger configuration—
15 points and 20 lines, with 3 points on each line
and 4 lines on each point.
This large Desargues configuration involves a third triangle,
needed for the proof (though not the statement ) of the
"if and only if" version of the theorem. Labeled simply
"Desargues' Theorem," the large configuration is the
frontispiece to Volume I (Foundations) of Baker's 6-volume
Principles of Geometry .
Point-line incidence in this larger configuration is,
as noted in a post of April 1, 2013, described concisely
by 20 Rosenhain tetrads (defined in 1905 by
R. W. H. T. Hudson in Kummer's Quartic Surface ).
The third triangle, within the larger configuration,
is pictured below.
The title "Space Trace" was suggested yesterday by Claude.ai.
How classical space leaves a Galois trace:
(Perspective Not as Symbolic Form)
From a post of June 8, 2014 —

See August 6, 2013 — Desargues via Galois.
For some backstory, see
http://m759.net/wordpress/?s=”I+Ching”+48+well .
See as well “elegantly packaged” in this journal.
“Well” in written Chinese is the hashtag symbol,
i.e., the framework of a 3×3 array.
My own favorite 3×3 array is the ABC subsquare
at lower right in the figure below —
The New York TImes reports this evening that
"Jon Underwood, Founder of Death Cafe Movement,"
died suddenly at 44 on June 27.
This journal on that date linked to a post titled "The Mystic Hexastigm."
A related remark on the complete 6-point from Sunday, April 28, 2013 —
(See, in Veblen and Young's 1910 Vol. I, exercise 11,
page 53: "A plane section of a 6-point in space can
be considered as 3 triangles perspective in pairs
from 3 collinear points with corresponding sides
meeting in 3 collinear points." This is the large
Desargues configuration. See Classical Geometry
in Light of Galois Geometry.)
This post was suggested, in part, by the philosophical ruminations
of Rosalind Krauss in her 2011 book Under Blue Cup . See
Sunday's post Perspective and Its Transections . (Any resemblance
to Freud's title Civilization and Its Discontents is purely coincidental.)
"Cézanne ignores the laws of classical perspective . . . ."
— Voorhies, James. “Paul Cézanne (1839–1906).”
In Heilbrunn Timeline of Art History . New York:
The Metropolitan Museum of Art, 2000–. (October 2004)
Some others do not.
This is what I called "the large Desargues configuration"
in posts of April 2013 and later.
The figure below is one approach to the exercise
posted here on December 10, 2016.

Some background from earlier posts —
Click the image below to enlarge it.
*The Hudson of the title is the author of Kummer's Quartic Surface (1905).
The Rosenhain of the title is the author for whom Hudson's 4×4 diagrams
of "Rosenhain tetrads" are named. For the "complexity to simplicity" of
the title, see Roger Fry in the previous post.
Images from Burkard Polster's Geometrical Picture Book —

See as well in this journal the large Desargues configuration, with
15 points and 20 lines instead of 10 points and 10 lines as above.
Exercise: Can the large Desargues configuration be formed
by adding 5 points and 10 lines to the above Polster model
of the small configuration in such a way as to preserve
the small-configuration model's striking symmetry?
(Note: The related figure below from May 21, 2014, is not
necessarily very helpful. Try the Wolfram Demonstrations
model, which requires a free player download.)
Labeling the Tetrahedral Model (Click to enlarge) —
Related folk etymology (see point a above) —
Related literature —
The concept of "fire in the center" at The New Yorker ,
issue dated December 12, 2016, on pages 38-39 in the
poem by Marsha de la O titled "A Natural History of Light."
Cézanne's Greetings.
"That in which space itself is contained" — Wallace Stevens
An image by Steven H. Cullinane from April 1, 2013:
The large Desargues configuration of Euclidean 3-space can be
mapped canonically to the 4×4 square of Galois geometry —
On an Auckland University of Technology thesis by Kate Cullinane —

The thesis reportedly won an Art Directors Club award on April 5, 2013.
See a search for "large Desargues configuration" in this journal.
The 6 Jan. 2015 preprint "Danzer's Configuration Revisited,"
by Boben, Gévay, and Pisanski, places this configuration,
which they call the Cayley-Salmon configuration , in the
interesting context of Pascal's Hexagrammum Mysticum .
They show how the Cayley-Salmon configuration is, in a sense,
dual to something they call the Steiner-Plücker configuration .
This duality appears implicitly in my note of April 26, 1986,
"Picturing the smallest projective 3-space." The six-sets at
the bottom of that note, together with Figures 3 and 4
of Boben et. al. , indicate how this works.

The duality was, as they note, previously described in 1898.


Related material on six-set geometry from the classical literature—
Baker, H. F., "Note II: On the Hexagrammum Mysticum of Pascal,"
in Principles of Geometry , Vol. II, Camb. U. Press, 1930, pp. 219-236
Richmond, H. W., "The Figure Formed from Six Points in Space of Four Dimensions,"
Mathematische Annalen (1900), Volume 53, Issue 1-2, pp 161-176
Richmond, H. W., "On the Figure of Six Points in Space of Four Dimensions,"
Quarterly Journal of Pure and Applied Mathematics , Vol. 31 (1900), pp. 125-160
Related material on six-set geometry from a more recent source —
Cullinane, Steven H., "Classical Geometry in Light of Galois Geometry," webpage
G. H. Hardy in A Mathematician's Apology —
|
What ‘purely aesthetic’ qualities can we distinguish in such theorems as Euclid’s or Pythagoras’s? I will not risk more than a few disjointed remarks. In both theorems (and in the theorems, of course, I include the proofs) there is a very high degree of unexpectedness, combined with inevitability and economy. The arguments take so odd and surprising a form; the weapons used seem so childishly simple when compared with the far-reaching results; but there is no escape from the conclusions. There are no complications of detail—one line of attack is enough in each case; and this is true too of the proofs of many much more difficult theorems, the full appreciation of which demands quite a high degree of technical proficiency. We do not want many ‘variations’ in the proof of a mathematical theorem: ‘enumeration of cases’, indeed, is one of the duller forms of mathematical argument. A mathematical proof should resemble a simple and clear-cut constellation, not a scattered cluster in the Milky Way. |
Related material:

"The Cardinal seemed a little preoccupied today."
See also a post found via a search in
this journal for "April 19 ".
Paradigms of Geometry:
Continuous and Discrete
The discovery of the incommensurability of a square’s
side with its diagonal contrasted a well-known discrete
length (the side) with a new continuous length (the diagonal).
The figures below illustrate a shift in the other direction.
The essential structure of the continuous configuration at
left is embodied in the discrete unit cells of the square at right.
See Desargues via Galois (August 6, 2013).

"The relevance of a geometric theorem is determined by what the theorem
tells us about space, and not by the eventual difficulty of the proof."
— Gian-Carlo Rota discussing the theorem of Desargues
What space tells us about the theorem :
In the simplest case of a projective space (as opposed to a plane ),
there are 15 points and 35 lines: 15 Göpel lines and 20 Rosenhain lines.*
The theorem of Desargues in this simplest case is essentially a symmetry
within the set of 20 Rosenhain lines. The symmetry, a reflection
about the main diagonal in the square model of this space, interchanges
10 horizontally oriented (row-based) lines with 10 corresponding
vertically oriented (column-based) lines.
Vide Classical Geometry in Light of Galois Geometry.
* Update of June 9: For a more traditional nomenclature, see (for instance)
R. Shaw, 1995. The "simplest case" link above was added to point out that
the two types of lines named are derived from a natural symplectic polarity
in the space. The square model of the space, apparently first described in
notes written in October and December, 1978, makes this polarity clearly visible:
From a recreational-mathematics weblog yesterday:
"This appears to be the arts section of the post,
so I’ll leave Martin Probert’s page on
The Survival, Origin and Mathematics of String Figures
here. I’ll be back to pick it up at the end. Maybe it’d like
to play with Steven H. Cullinane’s pages on the
Finite Geometry of the Square and Cube."
I doubt they would play well together.
Perhaps the offensive linking of the purely recreational topic
of string figures to my own work was suggested by the
string figures' resemblance to figures of projective geometry.
A pairing I prefer: Desargues and Galois —
For further details, see posts on Desargues and Galois.
(Continued from Mystery Box, Feb. 4, and Mystery Box II, Feb. 5.)
The Box

Inside the Box
Outside the Box
For the connection of the inside notation to the outside geometry,
see Desargues via Galois.
(For a related connection to curves and surfaces in the outside
geometry, see Hudson's classic Kummer's Quartic Surface and
Rosenhain and Göpel Tetrads in PG(3,2).)
The following excerpt from a January 20, 2013, preprint shows that
a Galois-geometry version of the large Desargues 154203 configuration,
although based on the nineteenth-century work of Galois* and of Fano,**
may at times have twenty-first-century applications.

Atkinson's paper does not use the square model of PG(3,2), which later
in 2013 provided a natural view of the large Desargues 154203 configuration.
See my own Classical Geometry in Light of Galois Geometry. Atkinson's
"subset of 20 lines" corresponds to 20 of the 80 Rosenhain tetrads
mentioned in that later article and pictured within 4×4 squares in Hudson's
1905 classic Kummer's Quartic Surface.
* E. Galois, definition of finite fields in "Sur la Théorie des Nombres,"
Bulletin des Sciences Mathématiques de M. Férussac,
Vol. 13, 1830, pp. 428-435.
** G. Fano, definition of PG(3,2) in "Sui Postulati Fondamentali…,"
Giornale di Matematiche, Vol. 30, 1892, pp. 106-132.
The following image gives a brief description
of the geometry discussed in last spring's
Classical Geometry in Light of Galois Geometry.

Update of Aug. 7, 2013: See also an expanded PDF version.
(Simplicity continued)
"Understanding a metaphor is like understanding a geometrical
truth. Features of various geometrical figures or of various contexts
are pulled into revealing alignment with one another by the
demonstration or the metaphor.
What is 'revealed' is not that the alignment is possible; rather,
that the alignment is possible reveals the presence of already-
existing shapes or correspondences that lay unnoticed. To 'see' a
proof or 'get' a metaphor is to experience the significance of the
correspondence for what the thing, concept, or figure is ."
— Jan Zwicky, Wisdom & Metaphor , page 36 (left)
Zwicky illustrates this with Plato's diamond figure
from the Meno on the facing page— her page 36 (right).
A more sophisticated geometrical figure—
Galois-geometry key to
Desargues' theorem:
| D | E | F | |
| S' | P | Q | R |
| S | P' | Q' | R' |
| O | P1 | Q1 | R1 |
For an explanation, see
Classical Geometry in Light of Galois Geometry.
This post was suggested by a search for the
Derridean phrase "necessary possibility"* that
led to web pages on a conference at Harvard
on Friday and Saturday, March 26**-27, 2010,
on Derrida and Religion .
The conference featured a talk titled
"The Poetics of the Broken Tablet."

I prefer the poetics of projective geometry.
An illustration— The restoration of the full
15-point "large" Desargues configuration in
place of the diminished 10-point Desargues
configuration that is usually discussed.
Click on the image for further details.
* See a discussion of this phrase in
the context of Brazilian religion.
** See also my own philosophical reflections
on Friday, March 26, 2010:
"You Can't Make This Stuff Up."
A Nested Sequence of Complete N-points and Their Sections
The complete space 6-point
(6 points in general position in space,
5 lines on each point, and 15 lines, 2 points on each)
has as a section
the large Desargues configuration
(15 points, 4 lines on each, and 20 lines, 3 points on each).
(Veblen and Young, Vol. 1, exercise 11, p. 53)
The large Desargues configuration may in turn be viewed as
the complete space 5-point
(5 points, 4 lines on each, and 10 lines, 2 points on each)
together with its section
the Desargues configuration
(10 points, 3 lines on each, and 10 lines, 3 points on each).
(Veblen and Young, Vol. I, pages 40-42)

The Desargues configuration may in turn be viewed as
the complete space 4-point (tetrahedron)
(4 points, 3 lines on each, and 6 lines, 2 points on each)
together with its section
the complete (plane) 4-side (complete quadrilateral)
(6 points, 2 lines on each, and 4 lines, 3 points on each).
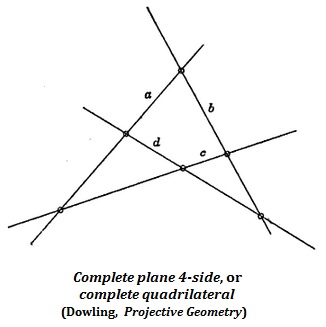
The complete quadrilateral may in turn be viewed as
the complete 3-point (triangle)
(3 points, 2 lines on each, and 3 lines, 2 points on each)
together with its section
the three-point line
(3 points, 1 line on each, and 1 line, 3 points on the line).
The three-point line may in turn be viewed as
the complete 2-point
(2 points, 1 line on each, and 1 line with 2 points on the line)
together with its section
the complete 1-point
(1 point and 0 lines).
Update of May 1: For related material, see the exercises at the end of Ch. II
in Veblen and Young's Projective Geometry, Vol. I (Ginn, 1910). For instance:

… And the history of geometry —
Desargues, Pascal, Brianchon and Galois
in the light of complete n-points in space.
(Rewritten for clarity at about 10 AM ET April 29, with quote from Dowling added.
Updated with a reference to a Veblen and Young exercise (on p. 53) on April 30.)


Veblen and Young, Projective Geometry, Vol. I ,
Ginn and Company, 1910, page 39:
"The Desargues configuration. A very important configuration
is obtained by taking the plane section of a complete space five-point."

Each of figures 14 and 15 above has 15 points and 20 lines.
The Desargues configuration within each figure is denoted by
10 white points and 10 solid lines, with 3 points on each line and
3 lines on each point. Black points and dashed lines indicate the
complete space five-point and lines connecting it to the plane section
containing the Desargues configuration.
In a 1915 University of Chicago doctoral thesis, Archibald Henderson
used a complete space six -point to construct a configuration of
15 points and 20 lines in the context not of Desargues ' theorem, but
rather of Brianchon 's theorem and of the Pascal hexagram.
Henderson's 1915 configuration is, it turns out, isomorphic to that of
the 15 points and 20 lines in the configuration constructed via a
complete space five -point five years earlier by Veblen and Young.
(See, in Veblen and Young's 1910 Vol. I, exercise 11, page 53:
"A plane section of a 6-point in space can be considered as
3 triangles perspective in pairs from 3 collinear points with
corresponding sides meeting in 3 collinear points." This is the
large Desargues configuration. See Classical Geometry in Light of
Galois Geometry.)
For this large Desargues configuration see April 19.
For Henderson's complete six –point, see The Six-Set (April 23).
That post ends with figures relating the large Desargues configuration
to the Galois geometry PG(3,2) that underlies the Curtis
Miracle Octad Generator and the large Mathieu group M24 —
See also Note on the MOG Correspondence from April 25, 2013.
That correspondence was also discussed in a note 28 years ago, on this date in 1985.
(Continued from December 31st, 2012)
"Principles before personalities." — AA saying
Art Principles
Part I:
Part II:
Baker's 1922 Principles of Geometry—
Art Personalities
"Of course, DeLillo being DeLillo,
it’s the deeper implications of the piece —
what it reveals about the nature of
film, perception and time — that detain him."
— Geoff Dyer, review of Point Omega
Related material:
A phrase of critic Robert Hughes,
"slow art," in this journal.
A search for that phrase yields the following
figure from a post on DeLillo of Oct. 12, 2011:

The above 3×3 grid is embedded in a
somewhat more sophisticated example
of conceptual art from April 1, 2013:
Update of April 12, 2013
The above key uses labels from the frontispiece
to Baker's 1922 Principles of Geometry, Vol. I ,
that shows a three-triangle version of Desargues's theorem.
A different figure, from a site at National Tsing Hua University,
shows the three triangles of Baker's figure more clearly:
Roberta Smith in 2011 on the American Folk Art Museum (see previous post):
"It could be argued that we need a museum of folk art
the way we need a museum of modern art,
to shine a very strong, undiluted light on
a very important achievement."
Some other aesthetic remarks:
"We have had a gutful of fast art and fast food.
What we need more of is slow art: art that holds time
as a vase holds water: art that grows out of modes
of perception and whose skill and doggedness
make you think and feel; art that isn't merely sensational,
that doesn't get its message across in 10 seconds,
that isn't falsely iconic, that hooks onto something
deep-running in our natures. In a word, art that is
the very opposite of mass media. For no spiritually
authentic art can beat mass media at their own game."
— Robert Hughes, speech of June 2, 2004,
quoted here June 15, 2007.
Perhaps, as well as museums of modern art and of folk art,
we need a Museum of Slow Art.
One possible exhibit, from this journal Monday:
The diagram on the left is from 1922. The 20 small squares at right
that each have 4 subsquares darkened were discussed, in a different
context, in 1905. They were re-illustrated, in a new context
(Galois geometry), in 1986. The "key" square, and the combined
illustration, is from April 1, 2013. For deeper background, see
Classical Geometry in Light of Galois Geometry.
Those who prefer faster art may consult Ten Years After.
The geometry posts of Sunday and Monday have been
placed in finitegeometry.org as
Classical Geometry in Light of Galois Geometry.
Some background:
See Baker, Principles of Geometry , Vol. II, Note I
(pp. 212-218)—
On Certain Elementary Configurations, and
on the Complete Figure for Pappus's Theorem
and Vol. II, Note II (pp. 219-236)—
On the Hexagrammum Mysticum of Pascal.
Monday's elucidation of Baker's Desargues-theorem figure
treats the figure as a 154203 configuration (15 points,
4 lines on each, and 20 lines, 3 points on each).
Such a treatment is by no means new. See Baker's notes
referred to above, and
"The Complete Pascal Figure Graphically Presented,"
a webpage by J. Chris Fisher and Norma Fuller.
What is new in the Monday Desargues post is the graphic
presentation of Baker's frontispiece figure using Galois geometry :
specifically, the diamond theorem square model of PG(3,2).
See also Cremona's kernel, or nocciolo :

Baker on Cremona's approach to Pascal—
"forming, in Cremona's phrase, the nocciolo of the whole."

A related nocciolo :
Click on the nocciolo for some
geometric background.
Background: Rosenhain and Göpel Tetrads in PG(3,2)
|
Introduction: The Large Desargues Configuration Added by Steven H. Cullinane on Friday, April 19, 2013 Desargues' theorem according to a standard textbook:
"If two triangles are perspective from a point The converse, from the same book:
"If two triangles are perspective from a line
Desargues' theorem according to Wikipedia
"Two triangles are in perspective axially [i.e., from a line]
A figure often used to illustrate the theorem,
A discussion of the "if and only if" version of the theorem
This large Desargues configuration involves a third triangle,
Point-line incidence in this larger configuration is,
The third triangle, within the larger configuration,
|

A connection discovered today (April 1, 2013)—
(Click to enlarge the image below.)
Update of April 18, 2013
Note that Baker's Desargues-theorem figure has three triangles,
ABC, A'B'C', A"B"C", instead of the two triangles that occur in
the statement of the theorem. The third triangle appears in the
course of proving, not just stating, the theorem (or, more precisely,
its converse). See, for instance, a note on a standard textbook for
further details.
(End of April 18, 2013 update.)
Update of April 14, 2013
See Baker's Proof (Edited for the Web) for a detailed explanation
of the above picture of Baker's Desargues-theorem frontispiece.
(End of April 14, 2013 update.)
Update of April 12, 2013
A different figure, from a site at National Tsing Hua University,
shows the three triangles of Baker's figure more clearly:
(End of update of April 12, 2013)
Update of April 13, 2013
Another in a series of figures illustrating
Desargues's theorem in light of Galois geometry:

See also the original Veblen-Young figure in context.
(End of update of April 13, 2013)

Rota's remarks, while perhaps not completely accurate, provide some context
for the above Desargues-Rosenhain connection. For some other context,
see the interplay in this journal between classical and finite geometry, i.e.
between Euclid and Galois.
For the recent context of the above finite-geometry version of Baker's Vol. I
frontispiece, see Sunday evening's finite-geometry version of Baker's Vol. IV
frontispiece, featuring the Göpel, rather than the Rosenhain, tetrads.
For a 1986 illustration of Göpel and Rosenhain tetrads (though not under
those names), see Picturing the Smallest Projective 3-Space.
In summary… the following classical-geometry figures
are closely related to the Galois geometry PG(3,2):
|
Volume I of Baker's Principles has a cover closely related to the Rosenhain tetrads in PG(3,2) |
Volume IV of Baker's Principles has a cover closely related to the Göpel tetrads in PG(3,2) |
|
Foundations (click to enlarge)
|
Higher Geometry (click to enlarge)
|
Powered by WordPress